Prevalence of disease

Crude and age-specific prevalence

Prevalence of disease is proportion, expressed as number of people with the outcome over total number of people in the community. For example, imagine a town T that has 400 inhabitants. 40 people in the town are diagnosed with influenza. Then, based on this definition, we will state that:

Prevalence of influenza in town T = (40 / 400) * 1000 or

100 per 1000 population

Crude prevalence helps to decide between two or more populations as to prevalence of diseases or health outcomes. For example,

• Town X has 40 cases of influenza

• Town Y has 50 cases of influenza

Apparently without knowing how many people live in each town, it may seem that Town X has more problem with influenza than town Y, but once you know the population in each town, you can understand the difference. Let's say, X has 400 people while town Y has 600 people. Then, we see that

• Influenza in Town X is 100 per 1000 population

• Influenza in Town Y is 83.3 per 1000 population

These information helps us to put the disease prevalence in right perspective.



Age specific prevalence

While crude prevalence is important, age-specific prevalence or prevalence specific to each age group is useful to get a picture of the problem. Consider again the stories of the two towns:

First, age-specific prevalence of diabetes among people in Town X



As you can see in this table, diabetes is absent among young children and as people age, the prevalence increases. This is shown in the following figure:





You can see that prevalence is high among the age group 55 years and above. Let's take the case of another town Y, and there too, we have diabetes health issue, and here are the table and figure for their prevalence:



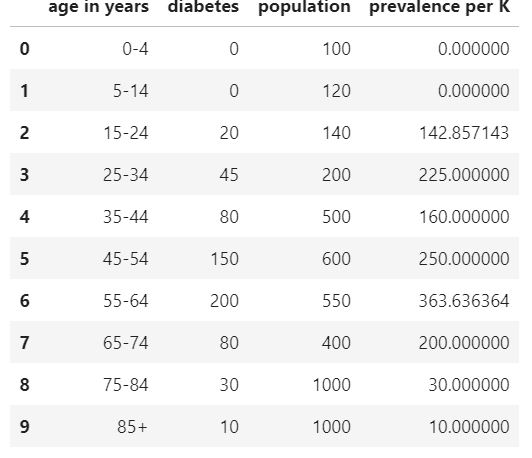
And the corresponding barplot shows that the age distribution of the prevalence of diabetes in this population of Town Y is different:



So, how can you compare the prevalence of diabetes in these two cities. Let's take a look at the crude rates:

• Crude prevalence of diabetes in town X: 125.31

• Crude prevalence of diabetes in town Y: 133.41

This tells us that overall, the prevalence of diabetes is higher in town Y than in town X. But this information tells us nothing about comparing the age-specific rates of diabetes we saw in both towns that are quite different. One way to compare the two towns would be to standardise on some other population and directly compare them. We will use such a population referred to as the SEGI world population. You will find the SEGI world population here:

https://www.who.int/healthinfo/paper31.pdf

We will use this population to standardise the rates, as follows:

Standardised prevalence and comparison

So, here's the SEGI world population from the PDF document I shared:

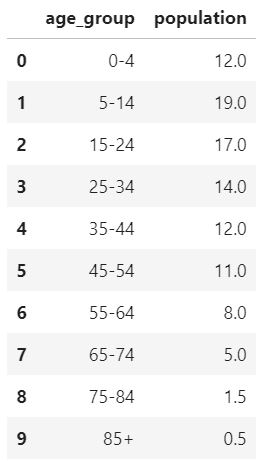
We will apply the prevalence we get from each of the town's X and Y, and we will see what numbers we get for the SEGI populations. So, our table now looks like this:

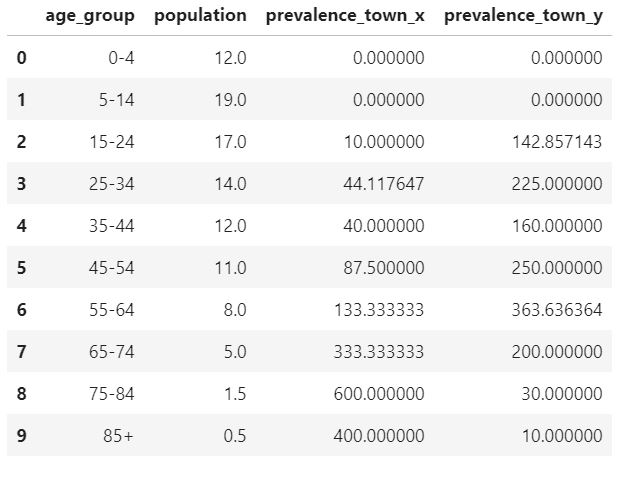
So, now we have a single table where we have age groups, we have the structure of the SEGI world population, and we also have the prevalence of diabetes in each of the two towns, X and Y. Now, if we multiply the prevalence of diabetes per age group in town X with the population in the same age band in SEGI world population, this will give us the total number of people with diabetes in the standard population, i.e., SEGI world population. We can continue this for each age band and we will end up with a table that looks like below:

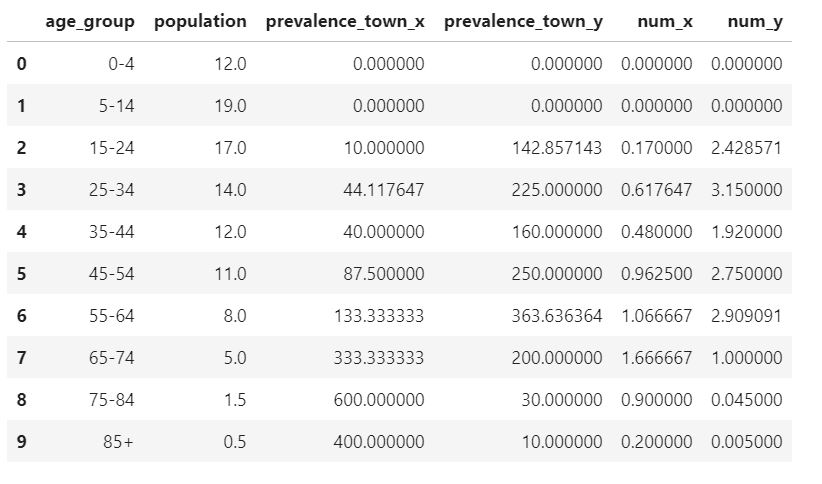
In the above table, note the columns num_x and num_y. In this table, “num_x” signifies the number of people in the corresponding age group in the SEGI population who would be diagnosed with Diabetes. Of course, you can say that 0.005 in the oldest age group 85+ does not make sense. But these are hypothetical “numbers” of people. It will soon make sense once we add these numbers up and remember that the total number of people in SEGI population add up to 100. So, let's add up the numbers and see what we get:

• For Town X, that number is: 6.06

• For Town Y, that number is: 14.20

Notice the difference in the magnitude of the crude prevalence? This is because now we have standardised our counts and we can now compare head to head what would be the morbidity comparison between diabetes cases in Town X versus in Town Y. This head to head comparison is referred to as Stanardised Morbidity and Mortality (where we count deaths) Ratio

In case of Town X and Town Y, we can say, the the risk of suffering from diabetes in Town Y compared with Town X is:

14.20 : 6.06 = 2.34



Summary

You just learned three key issues in the measurement of distribution of disease in epidemiology:

1. Prevalence as in crude prevalence. — Useful but hides the nuances that can be introduced when you have population difference across different groups of people

2. Age-specific prevalence. — When graphed, provides us with some indication as to which age groups have higher risk of diseases and which age groups have lower risk of diseases. This is useful information but you cannot use this information in head to head comparison of populations and groups of people.

3. Age-standardised prevalence. — Where we use a standardised population which can be used for head to head comparison between different populations.

We have used age groups here. You can use gender differences, ethnic differences and other points of comparison, you can even combine these various groups for standardisaion. In the end, the exact standardised population does not matter, what matters is the fact that such standardisation helps you to build a head to head comparison between different populations. You can use this to compare your own county or region with another part of the country in terms of health statistics, and indeed with the rest of the country from national statistics if you can get them.